Dynamical Systems
In mathematics, the study of dynamical systems investigates the evolution of a state under a deterministic update function. For instance, suppose you were given a number between zero and one and imagine it as a sequence of digits
Then an example of a dynamical system would at time step n to identify the nth digit. For example, the first few steps of the dynamical system corresponding to
would be 1,5,3,9,0,2,1... We can reference this particular dynamical system as E10, as it considers numbers expanded in base 10. One could equally choose a number between zero and one, consider its expansion in base 2, and then identify its digits. In any case, these dynamical systems exhibit what is known as a sensitive dependence on initial conditions. Loosely speaking, this means that a small perturbation in the initial number you are given will lead to significantly different dynamics. For example, if we were given random numbers identical up to the tenth decimal point, that is they differ by less than 0.0000000001, then up to the tenth iteration of E10 the dynamics would be the same. However, after the tenth iteration, the dynamics would diverge.
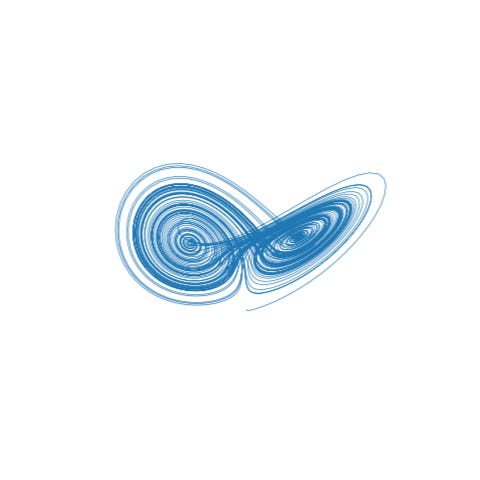
The dynamical system E10 is a discrete dynamical system as it evolves in steps. Continuous dynamical systems evolve along infinitesimally small steps. A prominent example of a continuous dynamical system is the Lorentz system,
Despite the Lorentz system also exhibiting sensitive dependence on initial conditions, under certain parameter values it exhibits an attractive behaviour. Its dynamics are concentrated on a near pre-determined structure. Although the exact manner in which the system evolves over the structure is sensitively dependent on the initial conditions, the global dynamics of the system are largely predictable.
The above are examples of deterministic dynamical systems, as the dynamics of the systems is completely determined by the set of initial conditions. This is because the evolution functions are deterministic functions. If instead one used a random evolution function then one would obtain a random dynamical system. For example, a random dynamical system may involve taking the previous state value, performing a deterministic manipulation of the value and adding random noise to the output.
Dynamical systems are ubiquitously used to model and investigate real-world processes. Of course, this requires an understanding of the governing equations of the dynamics of the process you intend to model. This a strong requirement that cannot always be met in practice, hence, the development of random dynamical systems. The randomness component is intended to model the complexity of real-world processes for which we have no set of governing equations. It is a fundamental question of physics as to whether natural processes are inherently random, and thus cannot be modelled by any deterministic dynamical system. Although quantum mechanics is probabilistic, it is still not clear whether quantum mechanics is the governing theory of the universe. It may be the case that we have developed a random theory to compensate for our lack of fundamental knowledge of the universe.
In any case, the theory of random dynamical systems is plentiful and has facilitated the modelling of many complex systems. In particular, one can make the argument that human civilisation is a random dynamical system. In which case we ask, is the dynamics of human civilisation configured such that its dynamics are constrained to an attractive surface?
If the answer is affirmative then there is a case to be made that the course of our history is pre-determined. An affirmative answer does not get rid of our free will as the exact trajectory over the attracting surface is still susceptible to perturbation, as we saw with the Lorentz system. What it does mean is that events in history are inevitable, it is just a matter of time as to when our civilisation will cross that region of the attracting surface.
History
The Rest of History Podcast pondered whether if any mildly conservative patriotic German leader would have been led down the same path as Hitler in a pre-World War Two era. It questions as to whether the forces of the random dynamical system overcome the desire of its individual components, making certain events inevitable. Perhaps, the exact events of history are not determined, but the global events are. For example, the exact trigger of World War Two is not pre-determined, but there was always going to be an eventual trigger due to the political tensions and geopolitical climate of the time.
Throughout history, we have often seen the same narrative, where a small set of individuals slowly amass into a gathering projecting an ideology. Eventually gaining sufficient power to enforce change and impose order. At a critical mass, the individuals can no longer effectively control the perception and implementation of their ideology. Leading to a sudden collapse of power, instilling chaos similar to that from which the embers of the original ideology emerged. The exact events governing this cycle vary due to a host of factors, however, the general narrative of the cycle remains largely intact.
There is something eerily Markovian about this cycle. Over successive generations that past is slowly forgotten, the lessons learned from previous tragedies dissipate, and society is left to commit the same mistakes. Consider the present climate, where rhetoric surrounding nuclear war is murmured in political discourse. After the Cold War, such murmurings have been strongly muted for the public were still recovering from the close calls. However, a large proportion of the society that vividly remembers those calls has moved on, leaving a naïve youth who have not experienced the trauma that such murmurings inflicted.
Therefore, in the Network State Balaji Srinivasan consistently argues for the importance of studying history. Balaji visualises this historical cycle as a cyclical staircase. That is, despite progressing along one dimension, say the technological dimension, the large-scale events of history are recurring. By studying history we ensure that we do not forget the lessons learned from past events, mitigating opportunities for mistakes to be repeated. However, we must be aware that we are not returning to the same point in history, we have evolved along several dimensions. Just as a staircase looks like a circle when viewed along one dimension, we need to carefully view history to make explicit the dimensions along which we are evolving. Understanding this will help us predict how the recurring events of history will contextualise in the modern era.
Indeed we have, from a quantitative perspective, better managed this cycle. In Better Angels of Our Nature, Steven Pinker demonstrates that across practically all metrics, we are better off than any previous society. More specifically, when metrics are averaged globally across time they all exhibit the same declining pattern. Of course, there are still local hotspots where violence spikes and points of civil instability. However, the severity of the localised regions of instability is also declining. The perceived severity of these local points in history is all relative to the current global climate. That is not to say that the suffering experienced in these localised regions is not a devastating human tragedy, it is just that in the larger context of human civilisation such suffering is becoming more seldom. Before we have mentioned that the Markovian nature of history occurs on the orders of generations, Steven Pinker argues that our memory of historical events in our own lifetime can be shrouded by misconceptions.
Steven Pinker's analysis shows that in general terms society is progressing in a positive direction. This is evidence to the fact that major historical cycles are prevalent, as their repetition offers opportunities for us to learn and better deal with the challenges when they reemerge. Consequently, their effects are less detrimental to society, facilitating our progress toward a more prosperous existence. Therefore, it is important we take the advice of Balaji and study history to maintain the lessons learned by previous generations, it is only by doing this that we can continue to progress as a civilisations. It is my worry that we begin to forget the lessons of the past and start suffering on a scale that inhibits societal progress.